Tomotaka Kuwahara
RIKEN Hakubi Team Leader, Analytical quantum complexity
RIKEN Hakubi Research Team
Outline
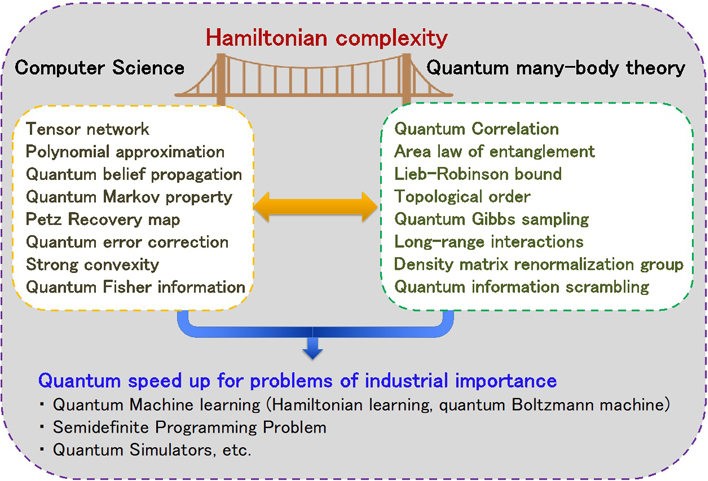
The field of revealing the computational complexity of quantum many-body system simulations when using a classical computer (or quantum computer) is called Hamiltonian Complexity. In particular, from the viewpoint of quantum supremacy of quantum computers and quantum algorithms employing NISQ (i.e., a small quantum computer without error correction), Hamiltonian complexity has become one of the most important research topics in the field of quantum information. Our research aims to solve open mathematical problems in Hamiltonian complexity.
Research Theme
・Entanglement area law
・Lieb-Robinson bound in interacting bosons
・Quantum Markov property at arbitrary temperatures
Representative Research Results
・ T. Kuwahara, K. Saito, “Strictly Linear Light Cones in Long-Range Interacting Systems of Arbitrary Dimensions,” Physical Review X, 10, 031010 (2020), Featured in Physics
RIKEN Research highlight: Long-range quantum interactions limit the speed of information
・T. Kuwahara, K. Saito, “Area law of noncritical ground states in 1D long-range interacting systems,” Nature Communications, 11 4478 (2020)
RIKEN Research highlight :Simplifying long-range quantum interactions in many-body systems
・T. Kuwahara, A. M. Alhambra, and A. Anshu, “Improved thermal area law and quasi-linear time algorithm for quantum Gibbs states,” Physical Review X, 11, 11047 (2021)
・A. Anshu, S. Arunachalam, T. Kuwahara, M. Soreimanifar (alphabet order), “Sample-efficient learning of quantum many-body systems,” Nature Physics, 17, 931–935 (2021), Featured in News&Views
RIKEN Research highlight: Determining the Hamiltonian of quantum systems with far fewer measurements
・T. Minato, K. Sugimoto, T. Kuwahara, “Fate of measurement-induced phase transition in long-range interactions ,” K. Saito, Physical Review Letters 128, 010603 (2022), Editor’ suggestion, Featured in Physics
・T. Kuwahara, K. Saito, “Exponential Clustering of Bipartite Quantum Entanglement at Arbitrary Temperatures,” To appear in Physical Review X